Question 1
Work out the value of each function.
1(a):
If f(x) = x -10, find f(20)
ANSWER: Simple Text Answer
Answer: 10
Workings:
Substitute x=20 into the equation
f(20)=20-10=10
Marks = 1
1(b):
If g(x)= \dfrac{-x}{4}, find g(-40)
ANSWER: Simple Text Answer
Answer: 10
Workings:
Substitute x=-40 into the equation
g(-40)=\dfrac{40}{4}=10
Marks = 1
1(c):
If f(x)=\dfrac{-x^2+10}{5}, find f(5)
ANSWER: Simple Text Answer
Answer: -3
Workings:
Substituting x=5 into the equation gives
f(5)=\dfrac{-5^2+10}{5}=\dfrac{-15}{5}=-3
Marks = 1
1(d):
If h(x)= -5(x -2)^2, find h(12)
ANSWER: Simple Text Answer
Answer: -500
Workings:
Substituting x=12 into the equation gives
h(12)=-5(12-2)^2 = -5\times 10^2=-5\times 100=-500
Marks = 1
1(e):
If g(x)=\dfrac{x-3}{4}, find g(83)
ANSWER: Simple Text Answer
Answer: 20
Workings:
Substituting x=83 into the equation gives
g(83)=\dfrac{83-3}{4}=\dfrac{80}{4}=20
Marks = 1
Question 2
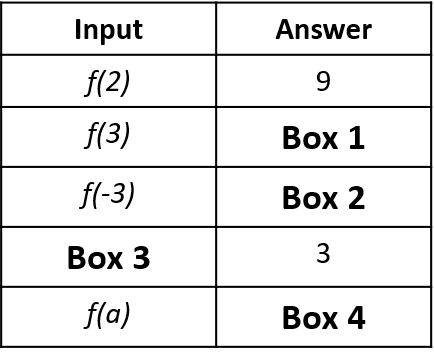
A function is given by f(x) = 3x+3
What are the values of boxes 1-4?
ANSWER: Multiple Choice (Type 1)
A: Box 1 = 12, Box 2 = -6, Box 3 = f(0), Box 4 = 3a+3
B: Box 1 = 15, Box 2 = -2, Box 3 = f(-1), Box 4 = a+3
C: Box 1 = 10, Box 2 = 1, Box 3 = f(3), Box 4 = a
D: Box 1 = 7, Box 2 = 0, Box 3 = f(1), Box 4 = a+1
Answer: A
Workings:
Box 1 = f(3)=3\times 3+3= 12
Box 2 = f(-3)=3\times-3 + 3=-6
Box 3 = 3x+3=3 so rearranging gives x=0
Box 4 = f(a)=3a+3
Marks = 2
Question 3
Considering the function f(x)=2x^2-1

3(a):
Using the table, what are the values for boxes 1 – 4?
ANSWER: Multiple Answers (Type 1)
Answer: Box 1 = 1, Box 2 = 21, Box 3 = 7, Box 4 = 1
Workings:
Box 1 = f(-1)=2\times (-1)^2-1=2\times 1-1=2-1 =1
Box 2 = f(4)=2\times 4^2-1=2\times 16-1=32-1=31
Box 3 = f(2) =2\times 2^2-1=2\times 4-1=2\times 4-1=8-1=7
Box 4 = f(1)=2\times 1^2-1=2\times 1-1=2-1=1
Marks = 1
3(b):
Using the table, what is the value of Box 5?
ANSWER: Multiple Choice (Type 1)
A: b^2-2
B: 3b^2+1
C: 2b^2-1
D: -b^2+2
Answer: C
Workings:
Box 5 = f(-b)=2\times (-b)^2-1=2\times b^2-1=2b^2-1
Marks = 1
Question 4
Given that f(x) = (x-5)^2, find:
4(a):
f(3)ANSWER: Simple Text Answer
Answer: 4
Workings:
f(3)=(3-5)^2=(-2)^2=4
Marks = 1
4(b):
f(0)ANSWER: Simple Text Answer
Answer: 25
Workings:
f(0)=(0-5)^2=(-5)^2=25
Marks = 1
4(c):
If f(x) = 0find the value of x
ANSWER: Simple Text Answer
Answer: x=5
Workings:
(x-5)^2=0
x-5=0
x=5
Marks = 2
Question 5
Solve the following functions.
5(a):
f(x) = x -10Solve f(x) = 0
ANSWER: Simple Text Answer
Answer: x=10
Workings:
f(x)=x-10=0
x=10
Marks = 1
5(b):
g(x)= \dfrac{-x}{4}Solve g(x) = 0.25
ANSWER: Simple Text Answer
Answer: x=-1
Workings:
g(x)=\dfrac{-x}{4}=0.25
-x=1
x=-1
Marks = 1
5(c):
f(x)=\dfrac{x^2+11}{5}Solve f(x)=12
ANSWER: Multiple Choice (Type 1)
A: \pm 4
B: \pm 5
C: \pm 6
D: \pm 7
Answer: D
Workings:
f(x) = \dfrac{x^2+11}{5}=12
x^2+11=60
x^2=49
x=\pm 7
Marks = 2
5(d):
h(x)=-5(x-2)^2For which values of x does h(x) = -5?
ANSWER: Multiple Answers (Type 2)
Answer: x=1 & x=3
Workings:
h(x)=-5(x-2)^2=-5
(x-2)^2=1
x-2=\pm 1
[latex]x=1 OR x=3
Marks = 2
