Question 1:

Question 1(a): [1 mark]
What is the gradient of the line f?
Answer type: Multiple choice type 2
A: y = 2x -2
B: y = 4x - 2
C: y = 2x + 4
D: y = 4x + 2
ANSWER: C: y = 2x + 4
WORKING: gradient =\dfrac{2}{1} = 2, intercept = 4
Question 1(b): [1 mark]
What is the gradient of the line g?
Answer type: Multiple choice type 2
A: y = 2x + \dfrac{3}{2}
B: y = -2x + \dfrac{3}{2}
C: y = 2x + 3
D: y = -2x + 3
ANSWER: D: y = -2x + 3
WORKING: gradient =\dfrac{- 2}{1} = - 2, intercept = 3
Question 1(c): [1 mark]
What is the gradient of the line h?
Answer type: Multiple choice type 2
A: y = x - 2
B: y = x + 2
C: y = - x -2
D: y = - x + 2
ANSWER: A: y = x - 2
WORKING: gradient =\dfrac{2}{2} = 1, intercept = -2.
Question 2(a): [2 marks]
Find the equation of the line AB where, A=(5,10) , B=(11,22).
Choose the correct answer.
Answer type: Multiple choice type 2
A: y = \dfrac{1}{2} x + \dfrac{15}{2}
B: y = 2x + 20
C: y = 2x
D: y = 2
ANSWER: C: y = 2x
WORKING:
m =\dfrac{\text{change in} \, y}{\text{change in} \, x} = \dfrac{22 - 10}{11 - 5} = \dfrac{12}{6} = 2.
y = 2x + c
Substituting in values of x and y gives
10 = 2(5) + c
10 = 10 + c
c = 0
y = 2x
Question 2(b): [2 marks]
Find the equation of the line CD where, A=(-2,-7) , B=(-14,-11).
Choose the correct answer.
Answer type: Multiple choice type 2
A: y = \dfrac{9}{8} x - \dfrac{19}{4}
B: y = 3x - 1
C: y = \dfrac{1}{3} x - \dfrac{19}{3}
D: y = \dfrac{1}{3} x - \dfrac{23}{3}
ANSWER: C: y = \dfrac{1}{3} x - \dfrac{19}{3}
WORKING:
m = \dfrac{\text{change in} \, y}{\text{change in} \, x} = \dfrac{-11 - \, - 7}{-14 - \, - 2} = \dfrac{-4}{-12} = \dfrac{1}{3}.
y = \dfrac{1}{3} x + c
Substituting in values of x and y gives
-7 = \dfrac{1}{3} (-2) + c
-7 = - \dfrac{2}{3} + c
c = - \dfrac{19}{3}
y = \dfrac{1}{3} x - \dfrac{19}{3}
Question 3:
In each of the following cases, find the gradient of the straight line by rearranging the equation.
Question 3(a): [2 marks]
y - 3 = 4(x - 2)
Answer type: Simple text answer
ANSWER: 4
WORKING:
y - 3 = 4(x - 2)
y - 3 = 4x - 8
y = 4x- 8 + 3
y = 4x - 5
m = 4
Question 3(b): [2 marks]
3y - 2 = 5x + 2
Answer type: Fraction
ANSWER: \dfrac{5}{3}
WORKING:
3y - 2 = 5x + 2
3y = 5x + 2 + 2
3y = 5x + 4
y = \dfrac{5}{3}x + \dfrac{4}{3}
m = \dfrac{5}{3}
Question 3(c): [2 marks]
\dfrac{2(3 - 5x)}{y} = 3
Answer type: Multiple choice type 2
A: - \dfrac{10}{3}
B: \dfrac{10}{3}
C: - \dfrac{3}{10}
D: \dfrac{3}{10}
ANSWER: A: - \dfrac{10}{3}
WORKING:
\dfrac{2(3 - 5x)}{y} = 3
3y = 2(3 - 5x)
3y = 6 - 10x
y = 2 - \dfrac{10}{3} x
m = - \dfrac{10}{3}
Question 4: [2 marks]
From the table below, find which 2 options out of 6 contain equations that have the same gradients.
Choose the correct answer below.
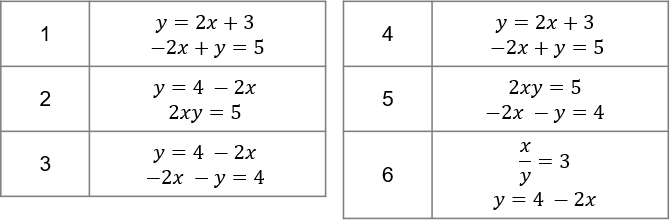
Answer type: Multiple choice type 2
A: 1 and 2
B: 2 and 5
C: 3 and 4
D: 3 and 6
ANSWER: C: 3 and 4
WORKING:
Option 3: y = 4 - 2x can be rearranged to y = -2x+4, so the gradient is -2.
-2x-y = 4 can be rearranged to y = -2x - 4, so the gradient is -2.
Option 4: y = 2x + 3 has a gradient of 2.
-2x+y=5 can be rearranged to y = 2x+5, so the gradient is 2.
Question 5:
The graph below is a distance time graph for a car, over 10 seconds, during a race.

Question 5(a): [2 marks]
Choose the correct equation for the straight line graph.
Answer type: Multiple choice type 2
A: y = 25x + 1075
B: y = 12.5x + 1075
C; y = 25x + 1750
D: y = 12.5x + 1750
ANSWER: B: y = 12.5x + 1075
WORKING:
m = \dfrac{\text{change in} \, y}{\text{change in} \, x} = \dfrac{25}{2} = 12.5
Question 5(b): [1 mark]
What does the value of m represent in terms of this car? Choose the correct answer.
Answer type: Multiple choice type 2
A: The distance the car travels.
B: The amount of time the car travels for.
C: The acceleration of the car.
D: The speed of the car.
ANSWER: D: The speed of the car.
WORKING: m is the change in distance over the change in time.
Question 6(a): [2 marks]
Two lines EF and GH are parallel.
EF: y = 5x - 2
G = (5,a) ; H = (2a,8)
Find the value of a.
Answer type: Simple text answer
ANSWER: a = 3
WORKING:
m = \dfrac{\text{change in} \, y}{\text{change in} \, x} = \dfrac{8 - a}{2a - 5} = 5
8 - a = 5(2a - 5)
8 - a = 10a - 25
11a = 33
a = 3
Question 6(b): [2 marks]
What is the correct equation of GH?
Answer type: Multiple choice type 2
A: y = 5x + 22
B: y = 5x + 28
C: y = 5x - 22
D: y = 5x - 28
ANSWER: C: y = 5x - 22
WORKING:
y = 5x + c
3 = 5(5) + c
3 = 25 + c
c = - 22
y = 5x - 22
