Question 1
Below is a list of shapes and their names.
Match each shape to the correct name.
The first one has been done for you.
(Please start each shape name with a capital)

ANSWER: Multiple Answers (Type 1)
Answer: B = Parallelogram, C = Pentagon, D = Rhombus, E = Hexagon, F = Trapezium
Workings:
Shape B has four sides and two pairs of sides that are parallel to each other. There are no lines of symmetry. Therefore it is a Parallelogram.
Shape C has 5 sides, so it is a Pentagon.
Shape D has 4 equal sides, 2 pairs of parallel sides, 2 pairs of equal angles and 2 lines of symmetry, so it is a Rhombus
Shape E has 6 sides, so it is a Hexagon.
Shape F has 4 sides and 1 pair of parallel sides, so it is a Trapezium.
Marks = 5
Question 2
Three irregular shapes are shown below:
2(a):
Choose the correct number of sides and the name of the following shape:

ANSWER: Multiple Choice (Type 2)
A: 5-sided Pentagon
B: 5-sided Hexagon
C: 6-sided Pentagon
D: 6-sided Hexagon
Answer: D
Workings:
Counting the sides tells us that there are 6 sides, meaning it is a Hexagon.
Marks = 1
2(b):
Choose the correct number of sides and the name of the following shape:

ANSWER: Multiple Choice (Type 2)
A: 6-sided Hexagon
B: 6-sided Heptagon
C: 7-sided Heptagon
D: 7-sided Hexagon
Answer: C
Workings:
Counting the sides tells us there are 7 sides, meaning it is a Heptagon
Marks = 1
2(c):
Choose the correct number of sides and the name of the following shape:
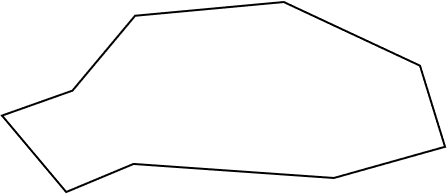
ANSWER: Multiple Choice (Type 2)
A: 7-sided Heptagon
B: 8-sided Octagon
C: 9-sided Nonagon
D: 10-sided Decagon
Answer: C
Workings:
Counting the sides tells us there are 9 sides, meaning it is a Nonagon.
Marks = 1
Question 3
The diagram below shows a garden.
All the corners meet at a right angle

Determine the area of the garden in m^2.
ANSWER: Simple Text Answer
Answer: 30
Workings:
We can split the diagram, as shown below, into two rectangles.

We know that the right-hand rectangle has sides 5m and 3m so the Area = 3\times 5=15 m^2
We can calculate side x by subtracting the 5m side of the right-hand rectangle from the bottom 8m side of the original shape.
This gives us 8-5 = 3 m=x
We can therefore calculate the area of the left-hand rectangle with 5x=5\times 3=15
Adding the two rectangles together gives us the total area.
15+15=30 m^2
Marks = 3
Question 4
An advertising company designs a poster in the shape of a parallelogram.
The client wants a bright background colour to be applied to the poster.

Calculate the area that needs to be painted in cm^2.
ANSWER: Simple Text Answer
Answer: 990
Workings:
The area of a Parallelogram is given by Base\times Height = Area
22\times 45=990 cm^2
Marks = 2
Question 5
The diagram below shows the a plan for the floor of a warehouse.

5(a):
Calculate the area of the warehouse floor in m^2.
ANSWER: Simple Text Answer
Answer: 33
Workings:
Area of a Trapezium is given by: Area = \dfrac{1}{2}\times (a+b) \times Height where a and b are the lengths of the parallel sides.
Area = \dfrac{1}{2}\times (9+13)\times 3 = 33
Marks = 3
5(b):
The warehouse owner has two identical warehouses.
How much would it cost to buy tiles to cover the floor of both warehouses in £, given that the tiles selected cost £25 per m^2?
ANSWER: Simple Text Answer
Answer: 1650
Workings:
We have two identical warehouses, so double the area of part (a)
2\times 33 = 66
Each m^2 cost £25, so to find the total cost we multiply the total area by the cost per m^2 tile.
66\times 25 = 1650
Marks = 2
Question 6
Consider the nets A, B, C and D.

6(a):
Which net will properly form a cube with a lid?
ANSWER: Multiple Choice (Type 1)
A: Net A
B: Net B
C: Net C
D: Net D
Answer: D
Workings:
This is the only net with the correct number of sides, 6, and whose sides won’t overlap at all.
Marks = 1
6(b):
Which of these is a reason why at least one of the other nets doesn’t form a cube?
ANSWER: Multiple Choice (Type 1)
A: Faces are different sizes
B: Faces form a pyramid
C: Not enough faces
D: Too many faces
Answer: D
Workings:
A cube has 6 faces and A & B both have 7, so we know neither of them can form cubes.
Marks = 1
Question 7
A farmer has a house that sits at the edge of a field, with all sides meeting at right angles.
The field has the following measurements.

7(a):
The farmer wants to know how much fencing he will need to surround the field.
Calculate the total length of fencing in m.
ANSWER: Simple Text Answer
Answer: 280
Workings:
Although there is a rectangle missing in the corner of the field. The length of fencing creating this dent is the same as it would be if it created a complete rectangle without the house.
This means we have two sides of length 40 and two sides of length 100.
Total Length = 2\times 40 + 2\times 100=280
Marks = 3
7(b):
If the fence costs £5 per metre, determine the cost of fencing the entire field in £.
ANSWER: Simple Text Answer
Answer: 1400
Workings:
To find the total cost we just need to multiply the total length by the cost per metre
280\times 5=1400
Marks = 2
