Question 1: [3 marks]
Consider the following triangle.
\angle BAC = 30 \degree
Find x.
Give your answer to 1 decimal place.
Answer type: Simple text answer
ANSWER: 3.0 cm
WORKING:
x^2 = 6^2 + 5^2 - 2(6)(5) \cos(30) = 9.0385...
x = \sqrt{9.0385...} = 3.0 cm (1 dp)
Question 2: [3 marks]
The diagram below shows a triangle.

Calculate the value of x.
Give your answer to 2 significant figures.
Answer type: Simple text answer
ANSWER: 88 \degree
WORKING:
\cos(x) = \dfrac{10^2 + 7^2 - 12^2}{2(10)(7)} = \dfrac{1}{28}
x = 88 \degree (2 sf)
Question 3:
OL and NL are planks leaning against a slope.
OM = 12 cm
LM = 6.5 cm
LN = 20 cm
\angle NLM = 49.5 \degree
\angle LMO \neq 90 \degree
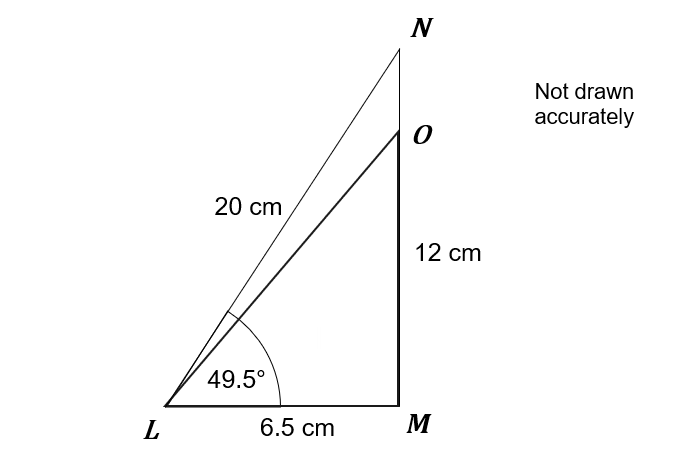
Question 3(a): [3 marks]
Find the length of the line ON.
Give your answer to 1 decimal place.
Answer type: Simple text answer
ANSWER: 4.5 cm
WORKING:
Let x = LM.
x^2 = 6.5^2 + 20^2 - 2(6.5)(20) \cos(49.5)
x = 16.5 (1 dp)
ON = 16.5 - 12 = 4.5 cm (1 dp)
Question 3(b): [3 marks]
Find the size of \angle LMN.
Give your answer to 1 decimal place.
Answer type: Simple text answer
ANSWER: 113.5 \degree
\cos (x) = \dfrac{16.5^2+6.5^2 - 20^2}{2(16.5)(6.5)} = -0.399
x = 113.5 \degree
Question 4:
At 16:00 hours, two ships depart from Port Phasmatys.
Ship A leaves on a bearing of 062\degree at 20 km per hour.
Ship B leaves on a bearing of 140 \degree at 12 km per hour.
Question 4(a): [1 mark]
How far is ship A from Port Phasmatys after 90 minutes?
Answer type: Simple text answer
ANSWER: 30 km
WORKING:
20 km in 60 minutes, so 30 km in 90 minutes.
Question 4(b): [3 marks]
Calculate the distance between A and B after 90 minutes.
Give your answer to the nearest kilometre.
Answer type: Simple text answer
ANSWER: 32 km
WORKING:
AB^2 = 30^2 + 18^2 - 2(30)(18) \cos(78) = 999.4454...AB = 32 km (nearest km)
Question 5:
The diagram below shows a triangle.

Question 5(a): [2 marks]
Using the cosine rule formula, we can find an expression in the form q^2 = p^2 - p + 61, where a is an integer.
Find the value of a.
Answer type: Simple text answer
ANSWER: 61
WORKING:
q^2 = (4+p)^2 + (5-p)^2 - 2(4+p)(5-p)\cos(120)
q^2 = 2p^2 - 2p + 41 + (4+p)(5-p) = p^2 - p + 61
Hence, a=61.
Question 5(b): [2 marks]
p is given to be 0.5. Find the value of q, to 1 decimal place.
Answer type: Simple text answer
ANSWER: 7.8
WORKING:
q^2 = 0.5^2 - 0.5 + 61 = 60.75q = \sqrt{60.75} = 7.8 (1 dp)
Question 6:
Consider the following triangle.

Question 6(a): [3 marks]
Using the cosine rule, find an expression in terms of x and y.
Choose the correct option from below.
Answer type: Multiple choice type 1
A: y^2 = x^2 + 3
B: y^2 = 3x^2 + 1
C: y^2 = 3x^2 + 3
D: y^2 = x^2 + 1
ANSWER: A: y^2 = x^2 + 3
WORKING:
y^2 = (x+1)^2 + (x-1)^2 - 2(x+1)(x-1) \cos(60)
y^2 = 2x^2 + 2 - (x^2-1) = x^2 + 3
Question 6(b): [1 mark]
x is an even number.
Determine whether y^2 must be odd or even.
Answer type: Multiple choice type 1
A: Odd
B: Even
ANSWER: A: Odd
WORKING:
x is even, so x^2 is even.
Hence, x^2+3 is odd, therefore y^2 is odd.
