Question 1
A class of 40 students measure their shoe sizes and summarise this information in a frequency table as shown.

1(a):
What is the modal shoe size?
ANSWER: Simple Text Answer
Answer: 5
Workings:
Shoe size 5 has the highest value in the frequency column so must be the modal shoe size.
Marks = 1
1(b):
What is the median shoe size?
ANSWER: Simple Text Answer
Answer: 5
Workings:
\dfrac{n+1}{2} = \dfrac{40+1}{2} = \dfrac{41}{2} = 20.5
Calculating the cumulative frequency: after shoe size 3, CF = 4. After shoe size 4, CF = 11. After shoe size 5, CF = 26.
The 20^{th} and 21^{st} values both fall in shoe size 5, so this is the median value.
Marks = 1
1(c):
Calculate the mean shoe size for the class to 3 decimal places.
ANSWER: Simple Text Answer
Answer: 5.025
Workings:
(3\times 4) + (4\times 7) + (5\times 15) + (6\times 12) + (7\times 2) = 12 + 28 + 75 + 72 + 14 = 201
\dfrac{201}{40} = 5.025
Marks = 2
Question 2
Data on the heights of 12 students has been collected.
150cm, 136cm, 142cm, 155cm, 160cm, 163cm
145cm, 139cm, 137cm, 154cm, 147cm, 149cm
2(a):

Using the data provided, find the values for a, b, c and d in the grouped frequency table.
ANSWER: Multiple Answers (Type 1)
Answers: a = 3, b=5, c=3, d=1
Workings:
136, 137 and 139 all fall into 130 < h \leq 140, giving a frequency of 3.
142, 145, 147, 149 and 150 all fall into 140 < h \leq 150, giving a frequency of 5.
154, 155 and 160 all fall into 150 < h \leq 160, giving a frequency of 3.
163 falls into 160 < h \leq 170, giving a frequency of 1.
Marks = 2
2(b):
What is the median height?
ANSWER: Multiple Choice (Type 2)
A: 130 < h \leq 140
B: 140 < h \leq 150
C: 150 < h \leq 160
D: 160 < h \leq 170
Answer: B
Workings:
\dfrac{n+1}{2} = \dfrac{12+1}{2} = \dfrac{13}{2} = 6.5
The 6^{th} and 7^{th} values both fall in the 140 < h \leq 150 category, so this is the median.
Marks = 1
2(c):
Calculate the mean height for the class to the nearest whole number.
ANSWER: Simple Text Answer
Answer: 148 cm
Workings:
Sum the values given at the beginning of the question to get 1777.
Divide by the number of values to get the mean.
\dfrac{1777}{12} = 148 cm to nearest whole number.
Marks = 1
Question 3
Data has been collected on the number of times 50 students exercise a week.
This has been summarised in the frequency table below.

3(a):
Calculate the mean number of times students exercise in a week.
ANSWER: Simple Text Answer
Answer: 2.9
Workings:
(1\times 5) + (2\times 16) + (3\times 15) + (4\times 7) + (5\times 7) = 145
\dfrac{145}{50} = 2.9
Marks = 2
3(b):
What is the median number of times a student exercises in a week?
ANSWER: Simple Text Answer
Answer: 33
Workings:
\dfrac{n+1}{2} = \dfrac{50+1}{2} = \dfrac{51}{2} = 25.5
The 25^{th} and 26^{th} values both have a value of 3, so this is the median.
Marks = 1
Question 4
A class of 35 students were asked how many extra toppings they like to have on pizza.
The data has been summarised in the frequency table below.
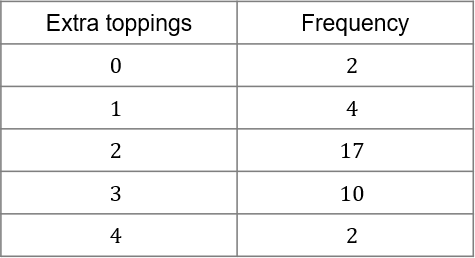
4(a):
Calculate the mean number of extra toppings taken.
ANSWER: Simple Text Answer
Answer: 2.17
Workings:
(0\times 2) + (1\times 4) + (2\times 17) + (3\times 10) + (4\times 2) = 4 + 34 + 30 + 8 = 76
\dfrac{76}{35} = 2.17
Marks = 2
4(b):
There are 910 students in the school.
Calculate an estimate for the number of students in the school who choose exactly one extra topping.
ANSWER: Simple Text Answer
Answer: 104
Workings:
910\times \dfrac{4}{35} = 104
Marks = 1
4(c):
Which of the following is an assumption that must be true for this to be a reliable estimate?
ANSWER: Multiple Choice (Type 1)
A: The school follows the same distribution as the class.
B: There are the same number of students in each class.
C: Everyone is asked on the same day.
D: The school has the same number of male as female students.
Answer: A
Workings:
In order for the answer to part (b) to be reliable, it must be that the data collected is representative of the entire school as well as the class.
Marks = 2
Question 5
Data on the time taken for 90 students to complete a 200m race has been summarised in the frequency table below.

What is the mean time taken to run the 200m race?
ANSWER: Simple Text Answer
Answer: 25.97
Workings:
(24\times 18) + (25\times 19) + (26\times 17) + (27\times 20) + (28\times 16) = 432 + 475 + 442 + 540 + 448 = 2337
\dfrac{2337}{90} = 25.97
Marks = 2
Question 6
The number of pairs of shoes owned by a class of 40 students has been collected and summarised in the frequency table below.
However, some of the data has been lost.

The mean of the data is 2.9.
Use this information to determine the missing frequencies in the table.
ANSWER: Multiple Answers (Type 1)
Answers: x=3, y=14
Workings:
3 + 8 + 12 + x + y = 40, x+y = 17\\
(x+(2\times 12)+(3y)+(4\times 8)+(5\times 3)) \div 40 = 2.9
x+3y = 45
Using the two simultaneous equations we find that
x=3 and y=14
Marks = 3
