Paper 2A
QUESTION 1 [2 marks]
A group of students were asked how many brothers and sisters they had. Their answers are recorded in the table below.

If a student is chosen at random, find the probability that the student will only have one sibling.
Give your answer as a fraction in its simplest form.
Answer type: Fraction
ANSWER: \dfrac{2}{5}
WORKING:
Total number of students = 4 + 12 + 8 + 3 + 1 + 2 = 30
Number of brother and sisters = 1
Number of students = 12
Probability = \dfrac{12}{30} = \dfrac{2}{5}
QUESTION 2 [1 mark]
Work out 600+1200\div300
Answer type: Simple text answer
ANSWER: 604
WORKING:
600 + 4 = 604
QUESTION 3 [1 mark]

Describe the nature of the correlation shown in the scatter graph.
Answer type: Multiple choice type 1
A: No correlation
B: Strong positive correlation
C: Strong negative correlation
D: Weak positive correlation
ANSWER: A
QUESTION 4 [2 marks]
What is \dfrac{5}{7}-\dfrac{1}{3}?
Answer type: Fraction
ANSWER: \dfrac{8}{21}
WORKING:
\dfrac{5}{7}-\dfrac{1}{3} = \dfrac{(5\times3)-(1\times7)}{7\times3} = \dfrac{15-7}{21} = \dfrac{8}{21}
QUESTION 5 [3 marks]
Samantha is investigating how many minutes it takes people to complete a puzzle.
She records their times below.
14, 17, 27, 11, 19, 14, 18, 13, 23, 25
Calculate the mean and range for this data set.
Answer type: Multiple answers type 1
ANSWER:
Mean = 18.1
Range = 16
WORKING:
Total = 14+12+27+11+19+14+18+13+23+25 = 181
Mean = 181 \div 10 = 18.1 minutes
Range = 27 - 11= 16 minutes
QUESTION 6 [2 marks]
Jack is making concrete using cement, sand and gravel in the ratio 1 : 2 : 4
He has: 39 kg cement, 112 kg sand and 204 kg gravel
What is the maximum amount of concrete that Jack can make?
Answer type: Simple text answer
ANSWER: 273 kg
WORKING:
Total number of parts =1+2+4=7
39 \times 7 = 273 kg
QUESTION 7
A bus company is looking to survey the age of its passengers. It decides to take a sample by recording the number of adult and children that are passengers on the same 8 am route on nine separate occasions.

Question 7(a) [1 mark]
Choose the correct scatter graph that plots the points from the table, along with the line of best fit.

Answer type: Multiple choice type 1
A:

B:

C:

D:

ANSWER: A
Question 7(b) [1 mark]
Does this data include any outliers? Choose the correct statement.
Answer type: Multiple choice type 1
A: Yes, (8,1)
B: Yes, (6,6)
C: Yes, (9,9)
D: No, there are no outliers
ANSWER: A
Question 7(c) [1 marks]
Using parts (a) and (b) or otherwise, estimate the number of children taking the bus, when there are 8 adults taking the bus.
Give your answer as a whole number.
Answer type: Simple text answer
ANSWER: 8
WORKING:

QUESTION 8 [1 mark]
Write the numbers in increasing order starting with the smallest.
10010,\,\,\,\, 11010,\,\,\,\, 10001, \,\,\,\, 11100, \,\,\,\, 11011
Answer type: Multiple choice type 1
A: 10001,\,\,\,\, 10010,\,\,\,\, 11010, \,\,\,\, 11011, \,\,\,\, 11100
B: 10001,\,\,\,\, 10010,\,\,\,\, 11100, \,\,\,\, 11010, \,\,\,\, 11011
C: 10001,\,\,\,\, 10010,\,\,\,\, 11011, \,\,\,\, 11010, \,\,\,\, 11100
D: 11100,\,\,\,\, 11011,\,\,\,\, 11010, \,\,\,\, 10010, \,\,\,\, 10001
ANSWER: A
Paper 2B
QUESTION 1 [2 marks]

Find the missing angle labelled A
Answer type: Simple text answer
ANSWER: 92 \degree
WORKING:
Angles in a triangle add up to 180\degree
180\degree - (41\degree + 47\degree) = 180\degree - 88\degree = 92\degree
QUESTION 2 [2 marks]
Megan is a florist.
She is arranging flowers for a display and has 16 roses, 8 daffodils and 13 tulips.
What is the probability of Megan randomly selecting a tulip to add to the display next?
Give your answer to three decimal places.
Answer type: Simple text answer
ANSWER: 0.351
WORKING:
\dfrac{13}{16+8+13} = 0.351 (3 dp)
QUESTION 3 [1 mark]

Choose the correct plan of the 3D shape.
Answer type: Multiple choice type 1
A: 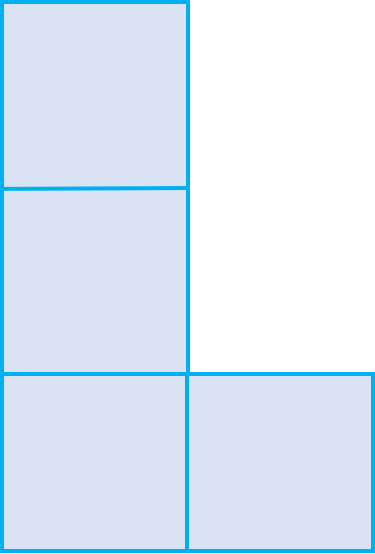 B:
B:  C:
C:  D:
D: 
ANSWER: A
QUESTION 4 [5 marks]
Three shops sell three different sized bottles of water.
Amazer shop sells water in 1 L bottles
Best-Value sells water in 50 cl bottles
Cheapest Buy sells 750 ml bottles of water

Using the three statements above, work out who got the cheapest water per litre.
Answer type: Multiple choice type 1
A: Jack
B: Sophie
C: Kabira
ANSWER: A
WORKING:
Jack: 4A + 5B +9C = 8.96
(4 \times 1) + (5 \times 0.5) + (9 \times 0.750) = 13.25 litres
\dfrac{8.96}{13.25} = £0.676 per litre
Sophie: 8A + 10B = 8.92
(8 \times 1) + (10 \times 0.5) = 13 litres
\dfrac{8.92}{13} = £0.686 per litre
Kabiria: 9A + 9B + 5C = 11.77
(9 \times 1) + (9 \times 0.5) + (5 \times 0.750) = 17.25 litres
\dfrac{11.77}{17.25} = £0.682 per litre
Jack bought the cheapest water per litre.
QUESTION 5 [4 marks]
The diagram shows a spherical glass paperweight with a radius, r, of 4 cm.

The density of glass is 8.23 g/cm^3.
Volume of a sphere = \dfrac{4}{3}\pi r^3
You are given that \pi = 3.14
Calculate the mass of the paperweight.
Give your answer to the nearest whole number.
Answer type: Simple text answer
ANSWER: 2205 g
WORKING:
Volume = \dfrac{4}{3} \times 3.14 \times 4^3 = 267.946... cm^3
Mass = 8.23 \times 267.946...=2205 g (nearest whole number)
QUESTION 6 [3 marks]
In 2010, the population of a town was 120000
The population of the town increased by 5 \% each year.
In 2012, \dfrac{1}{3} of the total population were children.
Calculate the population of children of the town in 2012
Answer type: Simple text answer
ANSWER: 44100
WORKING:
(2011) Year 1: 120000 \times 1.05 = 126000
(2012) Year 2: 126000 \times 1.05 = 132300
Population of children = \dfrac{1}{3} \times 132300 = 44100
QUESTION 7 [3 marks]
It takes 22 hours for a hosepipe with a flow of 12 litres per minute to fill a swimming pool.
How long will it take if its flow is reduced to 5 litres per minute?
Give your answer in hours.
Answer type: Simple text answer
ANSWER: 52.8 hours
ANSWER: A
WORKING:
22 hrs \times \, 720 litres per hr = \, 15840 litres needed
15840 litres \div \, 300 litres per hr = \, 52.8 hrs
QUESTION 8 [3 marks]
Last year Robert had two jobs. Robert worked:
in an office for 10 months and earned £1500 per month,
at a café for 15 weekends and earned £50 per weekend.
What fraction of his total income last year came from his work at the café?
Write the fraction in its simplest form.
Answer type: Fraction
ANSWER: \dfrac{1}{21}
WORKING:
Amount earned from working in the office: 10\times\text{£}1500 = \text{£}15000
Amount earned from the café: 15\times\text{£}50 = \text{£}750
Total income = £15750
\dfrac{750}{15750} = \dfrac{1}{21}
QUESTION 9
The table gives information about the types of drinks sold at a local café over the weekend.

Question 9(a) [3 marks]
Find the values of a, b, c, d and e from the two-way table above.
Answer type: Multiple answers type 1
ANSWER:
a = 105
b = 209
c = 82
d = 186
e = 387
WORKING:

Question 9(b) [1 mark]
Find the probability that a customer both enters the café on a Saturday and orders a coffee to 2 decimal places.
Answer type: Simple text answer
ANSWER: 0.27
WORKING:
Probability = \dfrac{104}{387}= 0.27 (2 dp)
QUESTION 10 [4 marks]
The diagram shows a plan of some cardboard packaging.
A company uses 400 of this type of packaging per day.
Calculate the total area of the cardboard used per day.

Answer type: Simple text answer
ANSWER: 44600 cm^2
WORKING:
Rectangle ABCD : Area =5\times7=35 cm^2
Rectangle CEFG : Area =6\times9=54 cm^2
Triangle DCG : Area = \frac{1}{2} \times 5\times9=22.5 cm^2
Area = 35+54+22.5=111.5 cm^2
Total area used per day = 111.5 \times 400 = 44600 cm^2
QUESTION 11 [3 marks]
Daniel cycles to university 5 days a week. He takes the same route every day and his journey to university takes 45 minutes at an average speed of 16 mph.
Given that he returns via the same route, determine how many miles he cycles in one week between home and university.
Answer type: Simple text answer
ANSWER: 120 miles
WORKING:
45 minutes = 0.75 hours
\text{distance}=\text{speed}\times\text{time} =16\times 0.75=12 miles
12\times 5 \times 2=120 miles cycled in one week
QUESTION 12 [2 marks]
Tom’s Grandma has £60 to give to her four grandchildren.
Tom gets \dfrac{1}{3} of the amount,
Alice gets 0.25 of the amount,
John gets 20\% of the amount
and Susan gets the rest.
Who receives the least amount of money?
Answer type: Multiple choice type 1
A: John
B: Tom
C: Susan
D: Alice
ANSWER: A
WORKING:
Tom =\text{£}60\div3=£20
Alice =\text{£}60\times0.25=£15
John =\text{£}60\times0.2=£12
Susan =\text{£}60-\text{£}20-\text{£}15-\text{£}12=£13
So John gets the least.
QUESTION 13 [2 marks]
The diagram shows the location of several towns.

Using the diagram, how much further is away is Town K from Town M than Town A from town J?
Answer type: Simple text answer
ANSWER: 20
A to J =4 squares =40 km
K to M =6 squares =60 km
Difference =60-40=20 km
Question 14 [4 marks]
Stephanie spent an average of 55 minutes watching TV from Monday to Friday.
On Saturday and Sunday, Stephanie spent H minutes watching TV each day.
The average for all 7 days of the week is 75 minutes.
Calculate the time Stephanie spends watching TV on Sunday. Give your answer in minutes.
You must show your workings.
Answer type: Simple text answer
ANSWER: 125 minutes
WORKING:
Total time: (55 \times 5)+2H = 275 +2H
Average time for the week: \dfrac{275+2H}{7}=75
The combined time for Saturday and Sunday: 2H =(75 \times 7) - 275 = 525 - 275 = 250
Time spent watching TV on Sunday: 250 \div 2 = 125 minutes
QUESTION 15 [3 marks]
Paula trains by running laps around an L-shaped field, as seen in the diagram below.

To reach her next goal she needs to run 5 km.
How many full laps does Paula need to run to achieve her goal?
Answer type: Simple text answer
ANSWER: 3 laps
WORKING:
Missing sides are \, 0.3-0.15=0.15 km and \, 0.6-0.2=0.4 km
Perimeter =0.2 + 0.15 + 0.4 + 0.15 + 0.6 + 0.3 = 1.8 km
Number of laps = 5 \div 1.8 = 2.77... laps = 3 full laps
