Paper 3A
QUESTION 1 [2 marks]
Work out 24-3^2\times2
Answer type: Simple text answer
ANSWER: 6
WORKING:
24 - 3^2\times2 = 24-9\times2=24-18=6
QUESTION 2 [2 marks]
What is \dfrac{8}{7} + \dfrac{6}{4}?
Give your answer as an improper fraction, in simplest form.
Answer type: Fraction
ANSWER: \dfrac{37}{14}
WORKING:
\dfrac{8\times4}{7\times4} + \dfrac{6\times7}{7\times4} = \dfrac{32}{28}+\dfrac{42}{28}= \dfrac{74}{28} = \dfrac{37}{14}
QUESTION 3 [1 mark]
Find the range of the following set of values
23, \,\, 11, \,\, 57, \,\, 113, \,\, 71, \,\, 5, \,\, 93
Answer type: Simple text answer
ANSWER: 108
WORKING:
Range = 113 - 5 = 108
QUESTION 4 [2 marks]
An object has volume 8000 cm^3 and a mass of 5 kg.
Find the density of this object, in g/cm^3.
Answer type: Simple text answer
ANSWER: 0.625 g/cm^3
WORKING:
5 kg = 5000 g
\text{Density} = 5000 \div 8000 = 0.625 g/cm^3
QUESTION 5 [1 mark]
If a pupil paid for lunch with two £1 coins, three 50p coins and two 20p coins how much was paid in total?
Answer type: Simple text answer
ANSWER: £3.90
2\times\text{£}1=\text{£}2,\,\, 3\times50\text{p} = \text{£}1.50,\,\,2\times20\text{p}=\text{£}0.40
Total =\text{£}2+\text{£}1.50+\text{£}0.40=\text{£}3.90
QUESTION 6 [3 marks]
It takes 5 people 3 and a half hours to decorate a room.
Assuming everyone works at the same rate, how long would it take for 2 people to decorate the same room?
Give your answer in hours.
Answer type: Simple text answer
ANSWER: 8.75 hours
WORKING:
5 people \times \, 3.5 hours = 17.5 hours of work
17.5 \div 2 = 8.75 hours for 2 people
QUESTION 7 [2 marks]
A student decides to raise money for charity by selling homemade lemonade for three weeks. The number of lemonade sales the student made each week are recorded in the bar chart below.

What percentage of the total lemonade sales were made in week 2?
Give your answer in simplest form.
Answer type: Simple text answer
ANSWER: 45 \%
WORKING:
Number of sales in week 2 =18
Total number of sales =10+18+12=40
\dfrac{18}{40}\times 100 = 45 \%
QUESTION 8 [3 marks]
A couple are doing their weekly fruit shop for their family. In their basket they have apples, oranges and bananas. There are 8 apples in the basket in total. If the ratio of apples in the basket to oranges in the basket is 2:3 and the ratio of oranges in the basket to bananas in the basket is 4:5, how many bananas are in the basket?
Answer type: Simple text answer
ANSWER: 15
WORKING:
(8\div2)\times3=12 (oranges in the basket)
(12\div4)\times5=15 (bananas in the basket)
PAPER 3B
QUESTION 1 [1 mark]
What are the coordinates of the point shown on this grid?

Answer type: Multiple choice type 1
A: (9,4)
B: (9,6)
C: (4,9)
D: (10,4)
ANSWER: A
QUESTION 2 [1 mark]

What is the name of the 3D shape that can be created with the net shown?
Answer type: Multiple choice type 1
A: Triangular based pyramid
B: Triangular prism
C: Frustum
D: Cylinder
ANSWER: A
QUESTION 3 [2 marks]
A football club sells 50 blue raffle tickets and 125 yellow raffle tickets.
Tickets are drawn randomly.
What is the probability that the first ticket drawn will be a blue ticket?
Give your answer as a decimal rounded to 3 decimal places.
Answer type: Simple text answer
ANSWER: 0.286
WORKING:
\dfrac{50}{50+125} = 0.286 (3 dp)
QUESTION 4 [3 marks]
The passenger list on a cruise ship shows that:
\dfrac{1}{10} of the passengers come from Italy.
\dfrac{1}{4} of the passengers come from Sweden.
And the other 520 passengers come from the UK.
How many passengers are there in total?
Answer type: Simple text answer
ANSWER: 800
WORKING:
1-\dfrac{4}{40}-\dfrac{10}{40}=\dfrac{26}{40} from the UK
\dfrac{520}{26} \times 40 = 20 \times 40 = 800
So, there are 800 passengers in total.
QUESTION 5
The diagram shows the floor plan to a storeroom.
The owner has ordered 14 items that each need at least 200 cm^2 of room.
CD = 15 cm, CA=45 cm, AB=100 cm, FE=15 cm, EB=10 cm

Question 5(a) [1 mark]
Is there enough room in the storeroom for all the items?
Answer type: Multiple choice type 1
A: Yes
B: No
ANSWER: B
Question 5(b) [4 marks]
By how much area is there spare/not enough of in the storeroom?
Answer type: Simple text answer
ANSWER: 50 cm^2
WORKING:
We can split the diagram into 2 rectangles and a triangle.
45\times 15=675 cm^2
85\times 10=850 cm^2
\frac{1}{2}\times 70\times 35=1225 cm^2
Total area =1225+675+850=2750 cm^2
Total area required =14\times200=2800 cm^2
There is not enough room by 2800 - 2750 = 50 cm^2
QUESTION 6 [5 marks]
Deborah’s height measures 4.8 ft
Deborah claims that she is taller than the mean height of her friends.
The height of her 13 friends are listed below.
141 cm, 130 cm, 135 cm, 144 cm, 130 cm, 152 cm, 145 cm,
140 cm, 153 cm, 160 cm, 180 cm, 120 cm, 134 cm
1 m = 3.28 ft
Is Deborah’s claim correct?
Choose the correct statement.
Answer type: Multiple choice type 2
A: Yes, her height is at least 1 cm more than the mean height of her friends.
B: Yes, her height is less than 1 cm more than the mean height of her friends.
C: No, her height is less than the mean height of her friends.
ANSWER: A
WORKING:
Total height of her friends = 1864 cm
Mean height of her friends = \dfrac{1864}{13} = 143.4 cm (1 dp)
Deborah’s height in m = 4.8 \div 3.28 = 1.463 m (3 dp)
Deborah’s height in cm 1.463 \times 100 = 146.3 cm (1 dp)
Deborah’s height is greater than the mean height of her friends, since 146.3 cm is greater than 143.4 cm
Therefore, Deborah is correct, and her height is at least 1 cm more than the mean height of her friends.
QUESTION 7 [3 marks]
This is a conversion graph between litres and pints.

A farmer sells pints of milk to a supermarket.
The supermarket pay 30p per litre for the milk.
On one day, the farmer sells 10500 pints of milk to the supermarket.
Using the conversion graph, find how much the supermarket pay for the milk on this day.
Answer type: Simple text answer
ANSWER: £2000
WORKING:
Using the conversion graph, 7 pints = 4 litres
10500 pints = (10500\div7)\times4=6000 litres
Price = 6000 \times 0.30 = £1800
QUESTION 8 [3 marks]
Harry puts £4000 in a bank account which earns 1.1\% compound return per year.
After 2 years, Harry uses the money in his bank account to buy a car worth £3500
Other than buying this car, he has not touched the bank account.
How much money will he have left in the bank account after he purchased the car?
Give your answer in pounds, to the nearest penny.
Answer type: Simple text answer
ANSWER: £588.48
ANSWER: A
WORKING:
Year 1: £4000 \times 1.011 = £4044
Year 2: £4044 \times 1.011 = £4088.484
Money remaining = £4088.484 - £3500 = £588.48 (nearest penny)
QUESTION 9 [3 marks]
Leslie is mixing paint to get a specific shade of purple.
He needs to make up 3 tins each of 1.5 L of paint.
To make the colour he mixes blue, red and white paint in the ratio 4 : 2 : 3
How much blue paint does Leslie need to complete the order?
Answer type: Simple text answer
ANSWER: 2 L
WORKING:
Total number of parts = 4+2+3 = 9
Total amount of paint = 3 \times 1.5 = 4.5 L
4.5 \div 9 = 0.5 L
0.5 \times 4 = 2 L of blue paint.
QUESTION 10 [5 marks]
Part of a circle is attached to a right angle triangle shown below.
CD = 6 cm,
CB = 12 cm,
AB = 13.4 cm.

You are given that \pi = 3.14
1 cm = 0.394 inches
Find the perimeter of the shape in inches.
Give your answer to 2 decimal places.
Answer type: Simple text answer
ANSWER: 16.08 inches
WORKING:
Length of arc CA = \dfrac{1}{4}\times3.14\times2\times6 = 9.42
Perimeter in cm =12+13.4+6+9.42=40.82 cm
Perimeter in inches = 40.82 \times 0.394 = 16.08 inches
Question 11 [4 marks]
Holly collects rare coins over the course of a year. She records the number of coins collected in the table below but accidentally misses out the number of £1 coins.
Holly wants to work out the median value of all the coins she collected in the year.
Given that the total value of the coins is £63.56
Work out the median coin value. You must show your workings.

Answer type: Multiple choice type 2
ANSWER: 20p
A: 20p
B: 10p
C: 50p
D:30p
WORKING:
Find the number of £1 coins by adding the values together:
0.12 + 0.14 + 0.50 + 2.10 + 2.20 +14.50 + x +38 =63.56 57.56+x=63.56 x=6
Now find the total number of coins:
12+7+10+21+11+29+6+19=115
Median is the \dfrac{115+1}{2}=58th coin.
Identifying the group that contains the 58th coin we find the median is 20p
QUESTION 12 [3 marks]
The diagram shows a block of wood.
A wood carver carves a shape out of this block of wood, such that only 55\% of the original block remains.
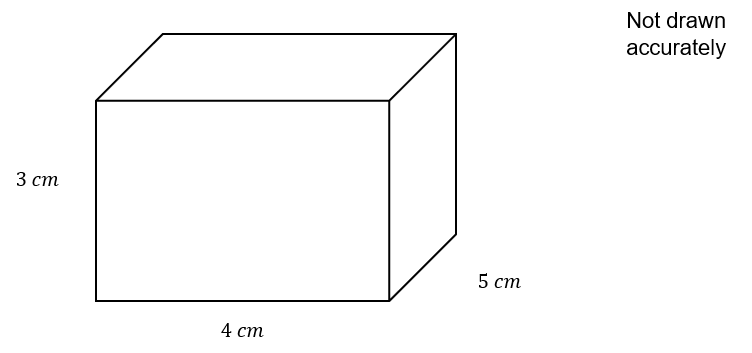
What is the volume of the carving?
Answer type: Simple text answer
ANSWER: 33 cm^3
WORKING:
Volume of block = 3 \times 4 \times 5 = 60 cm^3
Volume of carving = 60 \times 0.55 = 33 cm^3
QUESTION 13 [4 marks]
A shop has three offers on lemonade.

Which Offer provides the value for money?
Answer type: Multiple choice type 2
A: Offer 1
B: Offer 2
C: Offer 3
ANSWER: C: Offer 3
WORKING:
Offer 1:
New price = £1.90 \times 0.9 = £1.71
Price per litre = \dfrac{1.71}{2} = £0.855
Offer 2:
6 \times 330 = 1980 ml = 1.98 L
New price = £3.50 \div 2 = £1.75
Price per litre = \dfrac{1.75}{1.98} = £0.883
Offer 3:
600 ml = 0.6 L
New price = £0.75 \times \dfrac{2}{3} = £0.50
Price per litre = \dfrac{0.50}{0.6} = £0.833
Therefore, Offer 3 is the best value for money.
QUESTION 14 [2 marks]
The capacity of a cricket ground increases from 1900 to 3250
Calculate the percentage increase of the capacity of the cricket ground.
Give your answer as a percentage to 2 decimal places.
Answer type: Simple text answer
ANSWER: 71.05 \%
WORKING:
3250-1900=1350
\dfrac{1350}{1900}\times100 = 71.05\% (2 dp)
QUESTION 15 [1 mark]
The final grades achieved by 80 year 11 pupils in their summer biology and chemistry examinations have been recorded in the chart below.
What fraction of the students achieved a level 5 in biology? Give your answer in its lowest terms.

Answer type: Fraction
ANSWER: \dfrac{9}{40}
WORKING:
\dfrac{18}{40}=\dfrac{9}{40}