23. Quadratic Inequalities (Algebraically)

Question 1

LEVEL 8

Solve the inequality $x^2 \leq 81$.

Select the correct answer from the list below:

A: $x < -9 \text{ and } x > 9$

B: $x \leq -9 \text{ and } x \geq 9$

C: $- 9\leq x \leq 9$

D: $- 9 < x < 9$

CORRECT ANSWER:  C: $- 9\leq x \leq 9$

WORKED SOLUTION:

Square root both sides of the equation. We get $2$ square roots, positive and negative.

The range of values lie between and including $-9$ and $9$, so

Question 2

LEVEL 8

Solve the inequality $4 x^2 > 144$.

Select the correct answer from the list below:

A: $x < -6 \text{ and } x > 6$

B: $x \leq -6 \text{ and } x \geq 6$

C: $- 6\leq x \leq 6$

D: $- 6 < x < 6$

CORRECT ANSWER:  A: $x < -6 \text{ and } x > 6$

WORKED SOLUTION:

Square root both sides of the equation. We get $2$ square roots, positive and negative.

The range of values lie below $-6$ and above $6$, so

Question 3

LEVEL 8

Solve the inequality $x^2+7x+12<0$.

Select the correct answer from the list below:

A: $x<3\text{ and }x>4$

B: $3<x<4$

C: $-4<x<-3$

D: $x< -4\text{ and } x>-3$

CORRECT ANSWER:   C: $-4<x<-3$

WORKED SOLUTION:

To solve this inequality, we need to factorise the quadratic. Observing that $3\times 4=12$ and $3+4=7$, we get:

If we treat this like an equality to start off with, we can use this to plot the quadratic as $y=(x+3)(x+4)$. Note that the graph will cross the $x$-axis at $x=-4$ and $x=-3$.

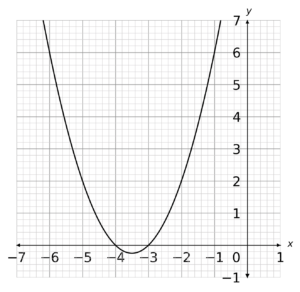
Now, we don’t want all these values we want all the ones that are $(x+3)(x+4)<0$. Because we plotted this as $y=(x+3)(x+4)$, we can say that $y<0$ and so only want the bit below the $y$-axis.

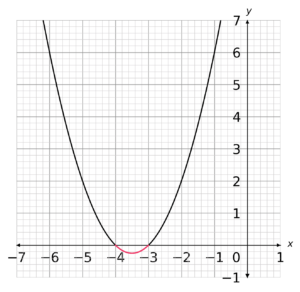
So, we can see that we only want the values of $x$ that are between $-4$ and $-3$. Therefore, the solution to the inequality is:

Question 4

LEVEL 8

Solve the inequality $x^2+3x-10\geq0$.

Select the correct answer from the list below:

A: $x\leq-5 \text{ and }x\geq2$

B: $-5<x<2$

C: $-5\leq x\leq2$

D: $x\leq-2 \text{ and }x\geq5$

CORRECT ANSWER:  A: $x\leq-5 \text{ and }x\geq2$

WORKED SOLUTION:

To solve this inequality, we need to factorise the quadratic. Observing that $(-2)\times5=10$ and $-2+5=3$, we get:

If we treat this like an equality to start off with, we can use this to plot the quadratic as $y=(x-2)(x+5)$. Note that the graph will cross the $x$-axis at $x=-5$ and $x=2$.

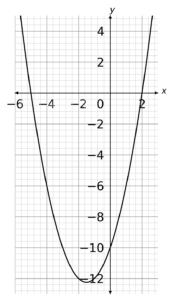
Now, we don’t want all these values we want all the ones that are $(x-2)(x+5)\geq0$. Because we plotted this as $y=(x-2)(x+5)$, we can say that $y\geq0$ and so only want the bits above the $y$-axis.

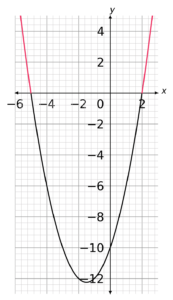
So, we can see that we only want the values of $x$ that are less than $-5$ and bigger than $2$. Therefore, the solution to the inequality is:

Question 5

LEVEL 8

Solve the inequality $x^2-4x>0$.

Select the correct answer from the list below:

A: $-2<x<2$

B: $x<-2 \text{ and }x>2$

C: $x<0 \text{ and }x>4$

D: $0<x<4$

CORRECT ANSWER:  C: $x<0 \text{ and }x>4$

WORKED SOLUTION:

To solve this inequality, we need to factorise the quadratic. Factorising here is pretty simple, as we can just pull out an $x$.

If we treat this like an equality to start off with, we can use this to plot the quadratic as $y=x(x-4)$. Note that the graph will cross the $x$-axis at $x=0$ and $x=4$.

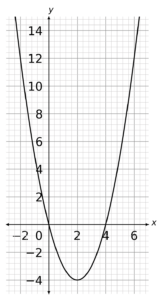
Now, we don’t want all these values we want all the ones that are $x(x-4)>0$. Because we plotted this as $y=x(x-4)$, we can say that $y>0$ and so only want the bits above the $y$-axis.

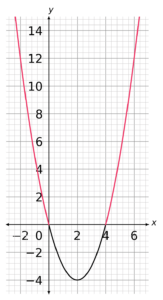
So, we can see that we only want the values of $x$ that are less than $0$ and bigger than $4$. Therefore, the solution to the inequality is: