NOTE: Q15-20 are the same as Q1-6 on June 17 Higher Paper 3
(DOESNT NEED CHECKING TWICE – CHANGE BOTH PAPERS IF THERE IS AN ERROR ON BOTH)
Question 1
The table below shows the heights of five Mountains in the United Kingdom.

Question 1(a) [1 mark]
Order the mountains in order of height, from lowest to highest.
Answer type: Multiple choice type 1
A: Whernside, Grasmoor, Scafell Pike, Ben Macdui, Ben Nevis
B: Ben Nevis, Ben Macdui, SCaffel Pike, Grasmoor, Whernside
C: Whernside, Grasmoor, Scafell Pike, Ben Nevis, Ben Macdui
D: Grasmoor, Whernside, Scafell Pike, Ben Macdui, Ben Nevis
ANSWER: A
WORKING:
736, 852, 978, 1309, 1345
Question 1(b) [1 mark]
Alex says “Ben Nevis is twice as tall as Whernside”
Is Alex correct?
Answer type: Multiple choice type 1
A: Yes
B: No
ANSWER: A
WORKING:
2 \times 736 = 1472 m, which is bigger than Ben Nevis, so Alex is incorrect.
Question 2 [2 marks]
Chocolates are sold in tubes and bags.
There are 10 chocolates per tube.
There are 25 chocolates per bag.
Ahmed buys t tubes of chocolate and b bags of chocolates.
Choose the correct expression, in terms of t and b, for the total number of chocolates Ahmed buys.
Answer type: Multiple choice type 1
A: 10t + 25b
B: 10b + 25t
C: 2t + 5b
D: 2b + 5t
ANSWER: A
Question 3
Below are five digits
4 \,\,\,\,\, 8 \,\,\,\,\, 5 \,\,\,\,\, 1 \,\,\,\,\, 2
Question 3(a) [1 mark]
Write down the greatest possible two digit number that can be made with two of the digits.
Answer type: Simple text answer
ANSWER: 85
Question 3(b) [1 mark]
Write down the three digit number closest to 500 that can be made with three of the digits.
Answer type:
ANSWER: 512
Question 4 [2 marks]
\dfrac{2}{3} of a number is 58
What is the number?
Answer type: Simple text answer
ANSWER: 87
WORKING:
(58 \div 2) \times 3 = 29 \times 3 = 87
Question 5
A kitchen floor is made from white tiles and black tiles.
\dfrac{1}{5} of the tiles are black.
Question 5(a) [1 mark]
What is the ratio of white tiles to black tiles?
Answer type: Multiple choice type 1
A: 4:1
B: 1:4
C: 5:1
D: 1:5
ANSWER: A
WORKING:
\dfrac{1}{5} are black, so \dfrac{4}{5} are white.
So, the ratio of white tiles to black is \dfrac{4}{5}:\dfrac{1}{5} which is 4:1
Question 5(b)
There are 40 tiles in total.
Calculate the number of white tiles.
Answer type: Simple text answer
ANSWER: 32
WORKING:
(40 \div 5) \times 4 = 8 \times 4 = 32
Question 6
Below is a list of numbers.
21 \,\,\,\,\, 17 \,\,\,\,\, 37 \,\,\,\,\, 25 \,\,\,\,\, 19 \,\,\,\,\, 30 \,\,\,\,\, 26
Question 6(a) [1 mark]
Calculate the median of the numbers in the list.
Answer type: Simple text answer
ANSWER: 25
WORKING:
Order the numbers from smallest to largest
17 \,\,\,\,\, 19 \,\,\,\,\, 21 \,\,\,\,\, 25 \,\,\,\,\, 26 \,\,\,\,\, 30 \,\,\,\,\, 37The median is the middle value, which is 25
Question 6(b) [2 marks]
Calculate the range of the numbers in the list.
Answer type:
ANSWER: 20
WORKING:
\text{Range} = \text{Largest value} - \text{Smallest value} = 37 - 17 = 20
Question 6(c) [2 marks]
Calculate the mean of the numbers in the list.
Answer type: Simple text answer
ANSWER: 25
WORKING:
Total = 17+19+21+25+26+30+37 = 175
Mean = \dfrac{175}{7} = 25
Question 7 [2 marks]
A football team plays two matches.
They can win, draw or lose each match.
How many possible outcomes are there?
Answer type: Simple text answer
ANSWER: 9
WORKING:
The possible combinations are:
WW, WD, WL, DW, DD, DL, LW, LD, LL
So, there are 9 in total
Question 8 [2 marks]
Jade wants to buy a new oven.
The oven costs £268
Jade will pay a £46 deposit.
She will then pay the rest of the cost in 6 equal monthly payments.
How much is each monthly payment?
Answer type: Simple text answer
ANSWER: £37
WORKING:
£268 - £46 = £222
£222 \div 6 = £37
Question 9 [3 marks]
Alfred is a butler.
The table shows information about the time it will take him to do chores, for Rodrick.

Alfred wants to do all four chores in one day.
Alfred starts at 10 am
He will have a 45 minute break during the day.
Rodrick arrives home from work at 5 pm.
Will Alfred have finished doing the chores by the time Rodrick arrives home?
Answer type: Multiple choice type 1
A: Yes
B: No
ANSWER: B
WORKING:
3 hours = 3 \times 60 = 180 minutes
1 \dfrac{1}{2} hours = 60 + 30 = 90 minutes
1 hour 20 minutes = 60 + 20 = 80 minutes
Total chore time = 180 + 90 + 80 + 40 = 390 minutes
Total time = 390 + 45 = 435 minutes = \dfrac{435}{60} = 7.25 hours = 7 hours 15 minutes
Finish time = 10:00 + 07:15 = 17:15 = 5:15 pm
So, Alfred will not finish his chores by the time Rodrick arrives home.
Question 10 [3 marks]
ABC is a straight line.
The length AB is 3 times the length of BC
AC = 76 mm
Calculate the length of AB
Answer type: Simple text answer
ANSWER: 57 mm
WORKING:
Ratio AB:BC = 3:1
Total number of parts = 3 + 1 = 4
1 part = 76 mm \div \, 4 = 19 mm
AB = 19 \times 3 = 57 mm
Question 11
P = 5q+8
Question 11(a) [2 marks]
Calculate the value of P when q = 3
Answer type: Simple text answer
ANSWER: 23
WORKING:
P = 5(3) + 8 = 15 + 8 = 23
Question 11(b) [2 marks]
Make q the subject of the formula P = 5q+8
Answer type: Multiple choice type 1
A: q = \dfrac{P-8}{5}
B: q = \dfrac{8-P}{5}
C: q = \dfrac{P-5}{8}
D: q = \dfrac{P+8}{5}
ANSWER: A
WORKING:
P = 5q+8
P - 8 = 5q
q = \dfrac{P-8}{5}
Question 12
The diagram below shows a cube of side length 3 cm

Question 12(a) [2 marks]
Calculate the volume of any solid made with 4 of these cubes.
Answer type: Simple text answer
ANSWER: 108 cm^3
WORKING:
Volume of cube = 3 \times 3 \times 3 = 27 cm^3
Volume of solid = 4 \times 27 = 108 cm^3
Question 12(b) [1 mark]
Choose the correct drawing of a possible cuboid made from all 4 of these cubes.
Answer type: Multiple choice type 1
A:

B:

C:

D:

ANSWER: A
Question 12(c) [2 marks]
Calculate the surface area of the correct drawing from part (b)
Answer type: Simple text answer
ANSWER: 144 cm^2
WORKING:
2 \times (6 \times 6) = 2 \times 36 = 72 cm^2
4 \times (6 \times 3) = 4 \times 18 = 72 cm^2
Surface area = 72 + 72 = 144 cm^2
Question 13 [5 marks]
The size of the largest angle in a triangle is 3 times the size of the smallest angle.
The other angle is 16 \degree less than the largest angle.
Calculate the size of each angle in the triangle.
Answer type: Multiple answers type 2 (can be either way round)
ANSWER:
angle 1 = 28 \degree
angle 2 = 68 \degree
angle 3 = 84 \degree
WORKING:
Let x be the smallest angle.
The the other angles are 3x and 3x-16 respectively.
We know that angles in a triangle add up to 180 \degree, so
x + 3x + (3x - 16) = 180
7x - 16 = 180
7x = 196
x = 28 \degree
Then, the other angles are 3 \times 28 = 84 \degree and 84 - 26 = 68 \degree respectively.
So, the three angles are 28 \degree, 68 \degree and 84 \degree
Question 14
Francis went on holiday to Australia.
Her flights cost a total of £1200
Francis stayed for 21 nights.
Her hotel room cost \$124 per night.
Francis paid for breakfast on 15 of the days.
Breakfast cost \$10 per day
The exchange rate was \$1.78 to £1
Question 14(a) [5 marks]
Calculate the total cost of the flights, the hotel and breakfast.
Give your answer in pounds, to the nearest penny.
Answer type: Simple text answer
ANSWER: £2747.19
WORKING:
Flights cost = £1200
Hotel cost in \$ = 21 \times \$124 = \$2604
Hotel cost in £ =\dfrac{2604}{1.78} = £1462.921...
Breakfast cost in \$ = 15 \times \$10 = \$150
Breakfast cost in £ = \dfrac{150}{1.78} = £84.269...
Total cost in £ = 1200 + 1462.921... + 84.269... = £2747.19 (nearest penny)
Question 14(b) [1 mark]
If there were more Australian dollars to £1, what effect would this have on the total cost, in pounds?
Answer type: Multiple choice type 1
A: The total cost would decrease
B: The total cost would increase
C: The total cost would stay the same
ANSWER: A
WORKING:
The flights would stay the same, but the rest would decrease, so it would decrease overall.
Question 15
\xi = \{ \text{even numbers less than } 20 \}
A = \{2, 6, 8, 12, 18 \}
B = \{2, 8, 16 \}
Question 15(a) [4 marks]
Choose the correct Venn diagram from the options below that represents this information.
Answer type: Multiple choice type 1
A:

B:

C:

D:

ANSWER: A
WORKING:
Missing numbers that are not in A nor B are \{4, 10, 14 \}
Question 15(b) [2 marks]
A number is chosen at random from the universal set, \xi.
What is the probability that the number is in the set A \cap B?
Give your answer as a fraction in its simplest form.
Answer type: Fraction
ANSWER: \dfrac{2}{9}
Question 16 [3 marks]
Solve the simultaneous equations
2x+3y = 8
4x-y=2
Answer type: Multiple answers type 1
ANSWER:
x = 1
y = 2
WORKING:
Let 2x+3y = 8 be equation 1,
and 4x-y=2 be equation 2.
Multiply equation 1 by 2:
4x+6y=16
Subtract equation 2 from equation the ‘new’ equation 1, so that the coefficients of x cancel:
(4x-4x) + (6y-(-y)) = 16-2
7y=14
y=2
Substitute y=2 into either equation, here we will put it into equation 2:
4x - 2 = 2
4x=4
x=1
Question 17
The table shows some information about the number of bedrooms 30 people have in their house.

Question 17(a) [1 mark]
Find the median number of bedrooms.
Answer type: Simple text answer
ANSWER: 3 bedrooms
WORKING:
Median is the between the 15th and 16th person, which is 3 bedrooms.
Question 17(b) [1 mark]
14 of the 30 people have 2 bathrooms in their house.
Mitch says that if you choose at random one of the 30 people, the probability that they have either 2 bathrooms or 2 bedrooms is \dfrac{22}{30} because
\dfrac{14}{30} + \dfrac{8}{30} = \dfrac{22}{30}
Is Mitch correct?
Answer type: Multiple choice type 1
A: Yes
B: No
ANSWER: B
WORKING:
No, because the number of bathrooms and number of bedrooms are not mutually exclusive – so addition is incorrect.
Question 18 [5 marks]
Nicola bakes 540 cookies.
She bakes only milk chocolate cookies, white chocolate cookies, triple chocolate cookies and raspberry cookies.
\dfrac{1}{3} of the cookies are milk chocolate.
15 \% of the cookies are white chocolate.
The ratio of the number of triple chocolate cookies to the number of raspberry cookies is 2:7
How many raspberry cookies does Nicola bake?
Answer type: Simple text answer
ANSWER: 217
WORKING:
\dfrac{1}{3} \times 540 = 180 milk chocolate cookies
0.15 \times 540 = 81 white chocolate cookies
There are 540 - 180 - 81 = 279 triple choc cookies and raspberry cookies
The ratio of triple chocolate cookies to the number of raspberry cookies is 2:7, so there are 9 parts
One part is worth 279 \div 9 = 31 cookies
The ratio of triple chocolate cookies to the number of raspberry cookies is therefore 62:217, by multiplying the ratio by 1 part (31)
Hence, Nicola bakes 217 raspberry cookies
Question 19 [4 marks]
In the diagram, AB, BC and CD are sides of a regular polygon P.

How many sides does polygon P have?
Answer type: Simple text answer
ANSWER: 15
WORKING:
The polygon shown in full is a decagon (10 sides)
\angle B = \dfrac{180}{10} (10-2) = 144 \degree
An equilateral triangle has all angles of 60 \degree
The interior angle of polygon P = 360 - 144 - 60 = 156 \degree
The exterior angle of polygon P = 180 - 156 = 24 \degree
The number of sides of polygon P = \dfrac{360}{24} = 15
Question 20 [4 marks]
The density of gin is 0.94 g/cm^3
The density of sugar syrup is 1.3 g/cm^3
The density of lime juice is 1.15 g/cm^3
60 cm^3 of gin is mixed with 20 cm^3 of sugar syrup and 30 cm^3 of lime juice to make a Southside cocktail drink, with a volume of 110 cm^3.
Work out the density of the drink.
Give your answer to 2 decimal places.
Answer type: Simple text answer
ANSWER: 1.06 g/cm^3
WORKING:
\text{density} = \text{mass} \div \text{volume}
Gin mass = 0.94 \times 60 = 56.4 g
Syrup mass = 1.3 \times 20 = 26 g
Lime juice mass = 1.15 \times 30 = 34.5 g
Total mass = 56.4 + 26 + 34.5 = 116.9 g
Total density = \dfrac{116.9}{110} = 106 g/cm^3 (2 dp)
Question 21 [2 marks]
Which two of the following triangles are mathematically similar?

Answer type: Multiple choice type 1
A: A and D
B: A and C
C: C and D
D: A and B
ANSWER: A
WORKING:
6 \div 1.5 = 4
8 \div 2 = 4
10 \div 2.5 = 4
The scale factor is the same for each side length from triangles A to triangle D.
Hence, triangles A and D are the same.
Question 22
Question 22(a) [2 marks]
Find the values of a,b,c,d and e from the table of values for y = \dfrac{3}{x}

Answer type: Multiple answers type 1
ANSWERS:
a = 6
b = 2
c = 1
d = 0.75
e = 0.6
WORKING:

Question 22(b) [2 marks]
Choose the correct plot of y = \dfrac{3}{x} for values of x from 0.5 to 5
Answer type: Multiple choice type 1
A:
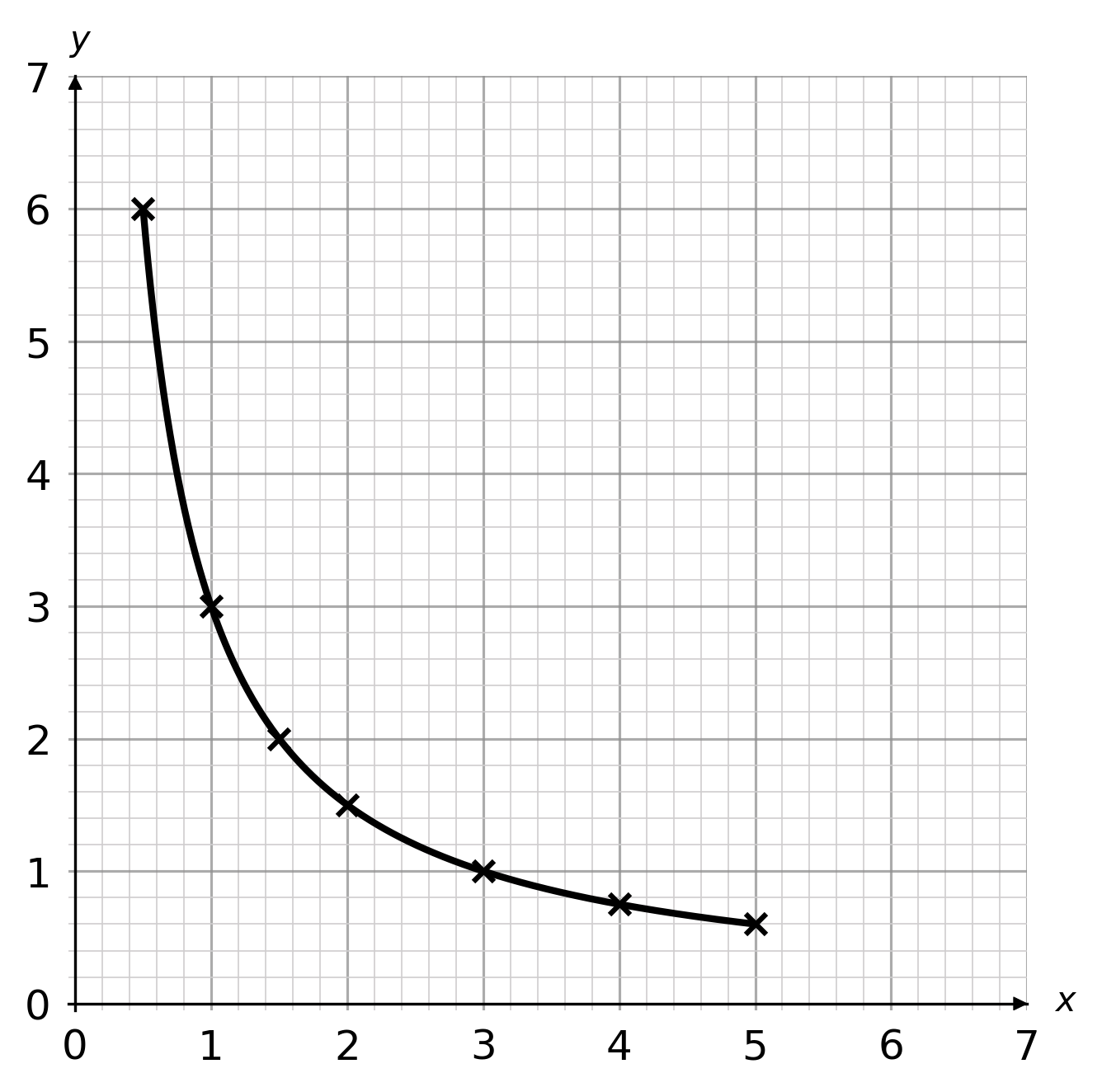
B:

C:

D:

ANSWER: A
Question 23
Gino owns a vintage car that has a value of £22000 correct to 2 significant figures.
Question 23(a) [1 mark]
What is the least possible value of the car?
Answer type: Simple text answer
ANSWER: £21500
Question 23(b) [1 mark]
What is the greatest possible value of the car?
Answer type: Multiple choice type 1
A: £22500
B: £23000
C: £22000
D: £22050
ANSWER: A
Question 23(c) [2 marks]
Gino has a second vintage car.
The value of this car increased by 10\%
His car then had a value of £16500
Calculate the value of the car before the increase.
Answer type: Simple text answer
ANSWER: £15000
WORKING:
£16500 \div 1.10 = £15000